| Relative Position, Velocity, and Acceleration in One Dimension |
| relative position | |||
| relative velocity | |||
| relative acceleration |
| xB/A | relative position of B with respect to A |
| vB/A | relative velocity of B with respect to A |
| aB/A | relative acceleration of B with respect to A |
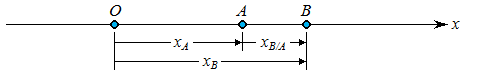
| (Eq1) |
|
| (Eq2) |
|
| (Eq3) |
|