Equations
|
| Instantaneous velocity |
| Average velocity |
Details
Consider the position P occupied by the particle at time t and displacement x in the next figure.
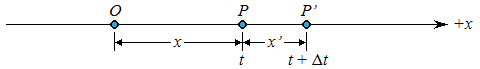
Consider also the position P' occupied by the particle at a later time t + Δt. The position coordinate of P' can be obtained by adding to x the displacement Δx, which will be positive or negative according to whether P' is to the right or to the left of P. The average velocity of the particle over the time interval Δt is defined as the quotient of the displacement Δx and the time interval Δt:
The instantaneous velocity u of the particle at the instant t is obtained from the average velocity by choosing shorter and shorter time intervals Δt and displacements Δx:
| Instantaneous velocity = u = | | |
Observing that the limit of the quotient is equal, by definition, to the derivative of x with respect to t:
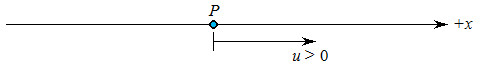
The velocity u is represented by an algebraic number which can be positive or negative. A positive value of u indicates that x increases, that is, that the particle moves in the positive direction as shown:
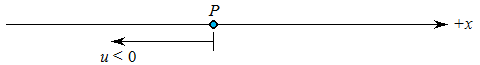
a negative value of u indicates that x decreases, that is, that the particle moves in the negative direction as shown:
The magnitude of u is known as the speed of the particle.


