---

Fracture Toughness

---

Quick
Fracture toughness measures the ability of a material containing a flaw to withstand an applied load. Fracture toughness is a quantitative property of the material.


Equation

(Eq1)     K = fσ√ πa

Stress intensity factor

Nomenclature

K	stress intensity factor
f	geometry factor for the specimen and flaw
σ	applied stress
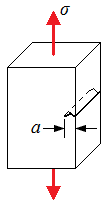
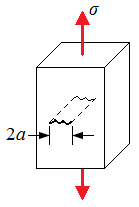
a	flaw size
Kc	fracture toughness
KIc	plain strain fracture toughness

Details

Fracture mechanics is the discipline concerned with the behavior of materials containing cracks or other small flaws. All materials, of course, contain some flaws. It may be desired to know the maximum stress that a material can withstand if it contains flaws of a certain size and geometry. Fracture toughness measures the ability of a material containing a flaw to withstand an applied load. Unlike the results of an impact test, fracture toughness is a quantitative property of the material.

A typical fracture toughness test may be performed by applying a tensile stress to a specimen prepared with a flaw of known size and geometry as shown:

Fracture toughness specimens

edge flaw	internal flaw

The stress applied to the material is intensified at the flaw, which acts as a stress raiser. For a simple test, the stress intensity factor, K, is:

(Eq1)

K = fσ√ πa

If the specimen is assumed to have an "infinite" width, the f ≅ 1.0.

By performing a test on a specimen with a known flaw size, the value of K that causes the flaw to grow and cause failure can be determined. This critical stress intensity factor is defined as the fracture toughness, K_c:

K_c = K required for a crack to propagate

Fracture toughness depends on the thickness of the sample: As thickness increase, fracture toughness K_c decreases to a constant value. This constant is called the plane strain fracture toughness K_Ic. It is K_Ic that is normally reported as the property of a material. There are tables that compare the value of K_Ic to the yield strength of various materials. There are also graphs that plot the fracture toughness versus the thickness of the specimen for different materials. Units for fracture toughness are:

ksi√

in.

=   1.0989 MPa√

m

The ability of a material to resist the growth of a crack depends on a large number of factors:
- Larger flaws reduce the permitted stress. Special manufacturing techniques, such as filtering impurities from liquid metals and hot pressing of particles to produce ceramic components, can reduce flaw size and improve fracture toughness.
- The ability of a material to deform is critical. For ductile metals the material near the tip of the flaw can deform, causing the tip of any crack to become blunt, reducing the stress intensity factor, and preventing growth of the crack. Increasing the strength of a given metal usually decreases ductility and gives a lower fracture toughness. Brittle materials such as ceramics and many polymers have much lower fracture toughnesses than metals.
- Thicker, more rigid materials have a lower fracture toughness than thin materials.
- Increasing the rate of application of the load, such as in an impact test, typically reduces the fracture toughness of the material.
- Increasing the temperature normally increases the fracture toughness, just as in the impact test.
- A small grain size normally improves fracture toughness, whereas more point defects and dislocations reduce fracture toughness. Thus, a fine-grained ceramic material may provide improved resistance to crack growth.