| Hydrostatic Forces on Submerged Plane Surfaces |
A plate exposed to a liquid, such as a gate valve in a dam, the wall of a liquid storage tank, or the hull of a ship at rest, is subjected to fluid pressure distributed over its surface. On a plane surface, the hydrostatic forces form a system of parallel forces, and the magnitude of the force and its point of application (center of pressure) often needs to be determined. In most cases, the other side of the plate is open to the atmosphere (such as the dry side of a gate), and thus atmospheric pressure acts on both sides of the plate, yielding a zero resultant. In such cases, it is convenient to subtract atmospheric pressure and use the gage pressure only as shown in Fig1 and Fig2.
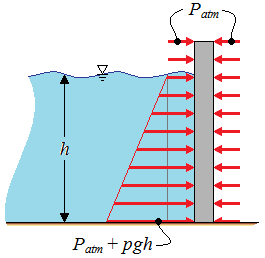
Fig1. Patm considered
| 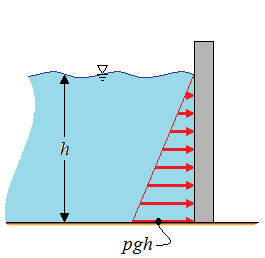
Fig2. Patm neglected
|
For example, Pgage = ρgh at the bottom of a body of water.
Consider the top surface of a flat plate of arbitrary shape completely submerged in a liquid, as shown:
The plane of this surface (normal to the page) intersects the horizontal free surface with an angle θ, and the line of intersection is the x axis. The absolute pressure above the liquid is P0, which is the local atmospheric pressure Patm if the liquid is open to the atmosphere (but P0 may be different than Patm if the space above the liquid is evacuated or pressurized). Then the absolute pressure at any point on the plate is:
P = P0 + ρgh = P0 + ρg y sin θ
where h is the vertical distance of the point from the free surface and y is the distance of the point from the x-axis (from point O in the figure). The resultant hydrostatic force FR acting on the surface is determined by integrating the force P dA acting on a differential area dA over the entire surface area:
| FR = | ∫ | | P dA = | ∫ | | (P0 + ρg y sin θ) dA = P0A + ρg sin θ | ∫ | | y dA |
But the first moment of area:
is related to the y-coordinate of the centroid (or center) of the surface by:
Substituting:
FR = (P0 + ρg yC sin θ)A = (P0 + ρghC)A = PCA = PaveA
where PC = P0 + ρghC is the pressure at the centroid of the surface, which is equivalent to the average pressure on the surface, and hC = yC sin θ is the vertical distance of the centroid from the free surface of the liquid as shown:
Thus, it may be concluded that the magnitude of the resultant force acting on a plane surface of a completely submerged plate in a homogeneous fluid is equal to the product of the pressure PC at the centroid of the surface and the area A of the surface as shown in the figure.
The pressure P0 is usually atmospheric pressure, which can be ignored in most cases since it acts on both sides of the plate. When this is not the case, a practical way of accounting for the contribution of P0 to the resultant force is simply to add an equivalent depth hequiv = P0/ρg to hC; that is, to assume the presence of an additional liquid layer of thickness hequiv on top of the liquid with absolute vacuum above.
Next the line of action of the resultant force FR must be determined. Two parallel force systems are equivalent if they have the same magnitude and the same moment about any point. The line of action of the resultant hydrostatic force, in general, does not pass through the centroid of the surface—it lies underneath where the pressure is higher. The point of intersection of the line of action of the resultant force and the surface is the center of pressure. The vertical location of the line of action is determined by equating the moment of the resultant force to the moment of the distributed pressure force about the x-axis. It gives:
| ypFR = | ∫ | | yP dA = | ∫ | | y(P0 + ρg y sin θ) dA = P0 | ∫ | | y dA + ρg sin θ | ∫ | | y 2 dA |
or:
yPFR = P0yCA + ρg sin θ Ixx, O
where yP is the distance of the center of pressure from the x-axis (point O in the figure) and:
is the second moment of area (also called the area moment of inertia) about the x-axis. The second moments of area are widely available for common shapes in engineering handbooks, but they are usually given about the axes passing through the centroid of the area. Fortunately, the second moments of area about two parallel axes are related to each other by the parallel axis theorem, which in this case is expressed as:
Ixx, O = Ixx, C + yC2A
where Ixx, C is the second moment of area about the x-axis passing through the centroid of the area and yC (the y-coordinate of the centroid) is the distance between the two parallel axes. Substituting the FR relation from Eq4 and the Ixx, O relation from Eq6 into Eq5 and solving for yP gives:
| yP = yC + | | Ixx, C | | [ yC + P0/(ρg sin θ)]A |
|
For P0 = 0, which is usually the case when the atmospheric pressure is ignored, it simplifies to:
Knowing yP, the vertical distance of the center of pressure from the free surface is determined from hP = yP sin θ.
The Ixx, C values for some common areas are given here. For these and other areas that possess symmetry about the y-axis, the center of pressure lies on the y-axis, directly below the centroid. The location of the center of pressure in such cases is simply the point on the surface of the vertical plane of symmetry at a distance hP from the free surface.
Pressure acts normal to the surface, and the hydrostatic forces acting on a flat plate of any shape form a volume whose base is the plate area and whose height is the linearly varying pressure, as shown:
This virtual pressure prism has an interesting physical interpretation: its volume is equal to the magnitude of the resultant hydrostatic force acting on the plate since V = ∫P dA, and the line of action of this force passes through the centroid of this homogeneous prism. The projection of the centroid on the plate is the pressure center. Therefore, with the concept of pressure prism, the problem of describing the resultant hydrostatic force on a plane surface reduces to finding the volume and the two coordinates of the centroid of this pressure prism.



